The Billiard Ball Example

Note, the alert observer will recognize that this analysis utilizes the conservation of momentum and the conservation of energy.
(For a review, see here and here and here and here and here, and take quiz 1 and quiz 2 and quiz 3 at the bottom.)

(An early draft of this work was posted online (July 2005) and can be found here and here.)

Experimental Evidence is the Truth Theory must Mimic.

Analysis of Collapse Time, for These Cases

Case 1. Free-fall from roof	Case 2. "collapse" every 10 floors	Case 3. "collapse" every floor	Case 4. "collapse" initiated ahead of "collapse" wave	Seismic Evidence

Bottom

Introduction

Very shortly after the events of September 11, 2001, the U.S. government proclaimed its certitude concerning who the attackers were -- 19 Arabs suicide bombers under the guidance of one Osama bin Laden. What followed in quick succession were ‘authoritative’ pronouncements, through NOVA and a few academicians, about what brought the WTC towers down. This early public authoritative consensus was that the buildings could not withstand the horrific onslaught of the plane crashes and subsequent fires. Since that time questions have arisen about the veracity of the Official Theory (as presented by government agencies) of the events of 9/11. One area of particular interest has been the issue of the WTC tower “collapses.” Are the given times possible?

The purpose of this site is to look at that question from a single, simple perspective -- that of the timing of those “collapses”. Absent other forces, gravity alone must have generated them. We will examine whether that was possible, from the perspective of the law of gravity and the visual record.

Note, the alert observer will recognize that this analysis utilizes the conservation of momentum. (For a review, see here and here and here and here and here.)

According to the "Official Story," how long did it take the WTC towers to collapse?

[Note: Dr. Wood is not establishing a "collapse" time, but is merely comparing the "official" value with an absolute minimum value.]

Page 305 of the 9/11 Commission Report states, "At 9:58:59, the South Tower collapsed in ten seconds, .... The building collapsed into itself, causing a ferocious windstorm and creating a massive debris cloud." (Chapter 9. html, pdf)

The August Fact Sheet (Answers to Frequently Asked Questions) by NIST states, "NIST estimated the elapsed times for the first exterior panels to strike the ground after the collapse initiated in each of the towers to be approximately 11 seconds for WTC 1 and approximately 9 seconds for WTC 2." (Question #6.)

The height of the South Tower (WTC2) is 1362 feet, and the height of the North Tower (WTC1) is 1368 feet, which are nearly the same.

Do these values seem reasonable? Let's calculate a few values we can use as a reference.

Other values of interest: (Note, these are only for reference and are not used as the "collapse" time.)

Columbia University's Seismology Group recorded seismic events of 10 seconds and 8 seconds in duration, which correspond to the collapses of WTC2 and WTC1, respectively.

Information Based on Seismic Waves recorded at Palisades New York

Seismology Group, Lamont-Doherty Earth Observatory, Columbia University

Event

origin time (EDT)
(hours:minutes:seconds)

Magnitude
(equivalent seismic)

Duration

Impact 1 at North Tower

08:46:26±1

0.9

12 seconds

Impact 2 at South Tower

09:02:54±2

0.7

6 seconds

Collapse 1, South Tower

09:59:04±1

2.1

10 seconds

Collapse 2, North Tower

10:28:31±1

2.3

8 seconds

For the following, I used the height of WTC1 as 1368 feet and considered each floor to be a height of 12.44 feet.
(1368/110 =12.44 ft/floor). I assumed gravity = 32.2 ft/sec² or 9.81 m/sec².

Simplicity

What can you prove with simple models of an enormously complex situation?

Let's say I tell you that I ran, by foot,
to a store (10 miles away), then
to the bank (5 more miles), then
to the dog track (7 more miles), then
to my friend's house (21 more miles), then home ...all in 2 minutes.

To disprove your story, I could present to you a simple case. I would present to you that the world's record for running just one mile is 3:43.13, or just under four minutes. So, it does not seem possible that I could have run over 40 miles in 2 minutes. i.e. It does not seem possible for me to have run 43 miles in half the time it would take the holder of the world's record to run just one mile. Even if you gave me the benefit of having run all 43 miles at world-record pace, it would not have been possible for me to have covered that distance in two minutes.

Remember, the proof need not be complicated. You don't need to prove exactly how long it should have taken me to run that distance. Nor do you need to prove how much longer it would have taken if I stopped to place a bet at the dog track. To disprove my story, you only need to show that the story I gave you is not physically possible.

Now, let us consider if any of those collapse times provided to us seem possible with the story we were given.

Case 1: Free-fall time of a billiard ball dropped from the roof of WTC1, in a vacuum Top

Let's consider the minimum time it would take the blue billiard ball to hit the pavement, more than 1/4 mile below (see below). Start the timer when the ball is dropped from the roof of WTC1. We'll assume this is in a vacuum, with no air resistance. (Note, large chunks of the building will have a very low surface area-to-mass ratio, so air resistance can be neglected.)

From the rooftop of WTC1, drop one (dark-blue) billiard ball over the edge. As it falls, it accelerates. If it were in a vacuum, it would hit the pavement, 1368 feet below, in 9.22 seconds, shown by the blue curve in the figure, below. It will take longer if air resistance is considered, but for simplicity, we'll neglect air resistance. This means that the calculated collapse times are more generous to the official story than they need to be.

(Click on image to enlarge.)

Figure 1. Minimum Time for a Billiard Ball dropped from the roof of WTC1 to hit the pavement below, assuming no air resistance.

Notice that the billiard ball begins to drop very slowly, then accelerates with the pull of gravity. If in a vacuum, the blue ball will hit the pavement, 1368 ft. below, 9.22 seconds after it is dropped. That is, unless it is propelled downward by explosives, it will take at least 9.22 seconds to reach the ground (assuming no air resistance).

Let's consider the "Pancake Theory"

According to the pancake theory, one floor fails and falls onto the floor below, causing it to fail and fall on the floor below that one, and so forth. The "pancake theory" implies that this continues all the way to the ground floor. In the case of both WTC towers, we didn't see the floors piled up when the event was all over, but rather a pulverization of the floors throughout the event. (see pictures below) So, clearly we cannot assume that the floors stacked up like pancakes. Looking at the data, we take the conservative approach that a falling floor initiates the fall of the one below, while itself becoming pulverized. In other words, when one floor impacts another, the small amount of kinetic energy from the falling floor is consumed (a) by pulverizing the floor and (b) by breaking free the next floor. In reality, there isn't enough kinetic energy to do either.[Trumpman][Hoffman] But, for the sake of evaluating the "collapse" time, we'll assume there was. After all, millions of people believe they saw the buildings "collapse."

Model A

Model B

(a): The floors remain intact and pile up like a stack of pancakes, from the top down.

(b): The floors blow up like an erupting volcano from the top down

(c): Note that the top "block" begins to disintegrate
before the damaged zone starts to move downward.

Video clip courtesy of 911review.org

Figure 2: Possibilities to consider for modeling the collapse.

Which of the two models, above, best matches the images below?

Top


(a) WTC2, demonstrating there is little to no free-fall debris ahead of the "collapse wave,"	(b) layer of uniform dust left by the "collapse."
Figure 3: Images from the "collapse."

If there was enough kinetic energy for pulverization, there will be pancaking or pulverization, but not both. For one thing, that energy can only be spent once. If the potential energy is used to pulverize a floor upward and outward, it can't also be used to accelerate the building downward. In order to have pancaking, a force is required to trigger the failure of the next floor. If the building above that floor has been pulverized, there can be no force pushing down. As observed in the pictures below, much of the material has been ejected upward and outward. Any pulverized material remaining over the footprint of the building will be suspended in the air and can't contribute to a downward force slamming onto the next floor. With pulverization, the small particles have a much larger surface-area-to-mass ratio and air resistance becomes significant. As we can recall, the dust took many days to settle out of the air, not hours or minutes. So, even though the mechanism to trigger the "pancaking" of each floor seems to elude us, let's consider the time we would expect for such a collapse.

(a)

(b)

(c)

(d)

Figure 4. Images illustrating what really happened that day.

To illustrate the timing for this domino effect, we will use a sequence of falling billiard balls, where each billiard ball triggers the release of the next billiard ball in the sequence by simply passing it in space. This is analogous to assuming pulverization is instantaneous and does not slow down the process. In reality, this pulverization would slow down the "pancake" progression, so longer times would be expected. Thus, if anything, this means the calculated collapse times are more generous to the official story than they need to be. Billiard balls are used as timing devices because they are identical (same size, mass, surface, aerodynamic properties...), all except for color. (The cue ball is not used in this example because it is a different size.)

Top

Case 2: ‘Progressive Collapse’ in ten-floor intervals

To account for the damaged zone, let’s simulate the floor beams collapsing every 10th floor, as if something has destroyed 9 out of every 10 floors for the entire height of the building. This assumes there is no resistance within each 10-floor interval. i.e. We use the conservative approach that there is no resistance between floor impacts. In reality there is, which would slow the collapse time further. Also, there was only damage in one 10-floor interval, not the entire height of the building. Thus, if anything, this means the calculated collapse times are more generous to the official story than they need to be. Refer to the figure below.

The clock starts when the blue ball is dropped from the roof (110th floor). Just as the blue ball passes the 100th floor, the red ball drops from the 100th floor. When the red ball passes the 90th floor, the orange ball drops from the 90th floor, ... etc. Notice that the red ball (at floor 100) cannot begin moving until the blue ball reaches that level, which is 2.8 seconds after the blue ball begins to drop.

This approximates the "pancaking" theory, assuming that each floor within the "pancaking" (collapsing) interval provides no resistance at all. With this theory, no floor below the "pancake" can begin to move until the progressive collapse has reached that level. For example, there is no reason for the 20th floor to suddenly collapse before it is damaged.

With this model, a minimum of 30.6 seconds is required for the roof to hit the ground. Of course it would take longer if accounting for air resistance. It would take longer if accounting for the structure's resistance that allows pulverization. The columns at each level would be expected to absorb a great deal of the energy of the falling floors. Thus, if anything, this means the calculated collapse times are more generous to the official story than they need to be.

(Click on image to enlarge.)

Figure 5. Minimum time for the collapse, if nine of every ten floors have been demolished prior to the "collapse."

Case 4: ‘Progressive Collapse’ at near free-fall speed

Now, consider the chart below.

(Click on image to enlarge.)

Figure 7. Minimum Time for a Billiard Ball dropped from the roof of WTC1 to hit the pavement below, assuming no air resistance.

Let's say that we want to bring down the entire building in the time it takes for free-fall of the top floor of WTC1. (Use 9.22 seconds as the time it would take the blue ball to drop from the roof to the street below, in a vacuum.) So, If the entire building is to be on the ground in 9.22 seconds, the floors below the "pancaking" must start moving before the "pancaking" ("progressive collapse”) reaches that floor, below. To illustrate this, use the concept of the billiard balls. If the red ball (dropped from the 100th floor) is to reach the ground at the same time as the blue ball (dropped from the 110th floor), the red ball must be dropped 0.429 seconds after the blue ball is dropped. But, the blue ball will take 2.8 seconds after it is dropped, just to reach the 100th floor in free fall. So, the red ball needs to begin moving 2.4 seconds before the blue ball arrives to "trigger" the red ball's motion. That is, each of these floors will need a 2.4 second head start for falling -- before the "free falling" floor is triggered to drop. But this also creates yet another problem: "the resistance paradox."[] How can the upper floor be destroyed by slamming into a lower floor if the lower floor has already moved out of the way?

Case 2, above, shows the red ball being dropped just as the blue ball passes that point.

Remember, I'm assuming the building was turning to dust as the collapse progressed, which is essentially what happened.

So, for the building to be collapsed in about 10 seconds, the lower floors would have to start moving before the upper floors could reach them by gravity alone.

Did we see this? I believe it's pretty clear in some of the videos. The "wave" of collapse, progressing down the building, is moving faster than free-fall speed. This would require something like a detonation or destruction sequence.

Realizing that, for example, the 40th floor needs to start moving before any of the upper floors have "free-fallen" to that point, why would it start moving? There was no fire there. And, if anything, there is less load on that floor as the upper floors turn to dust.

In the picture (at right), notice that WTC2 is less than half of its original height, yet has no debris that has fallen ahead of the demolition wave.

Figure 8. WTC2, demonstrating there is little to no free-fall debris ahead of the "collapse wave."

Top

So, how could the ground rumble for only 8 seconds while WTC1 "disappeared?"

I don't think this part of the building made a thud when it hit the ground.

Figure 9(a). Dust from "collapse."

This part of the building surely took a lot longer to hit the ground as dust than it would have if it came down as larger pieces of material. We know that sheets of paper have a very high surface-area-to-mass ratio and will stay aloft for long periods of time, which is why paper is an excellent material for making toy airplanes. The alert observer will notice that much of the paper is covered with dust, indicating that this dust reached the ground after the paper did. In the above picture, there are a few tire tracks through the dust, but not many, so it was probably taken shortly after one (or both) of the towers were down. Also, the people in the picture look like they've just come out of hiding, curious to see what just happened and to take pictures. If there had been a strong wind blowing the dust around, it would blow the paper away before it would have blown the dust onto the paper. So, the fact that much of the randomly-oriented paper is covered with dust indicates the relative aerodynamic properties of this dust.

Also, notice the dark sky as well as the haze in the distance. This was a clear day with no clouds in the sky... except for the dust clouds. This overcast appearance as well as the distant haze can only be explained by dust from the "collapse" that is still suspended in the air.

In a conventional controlled-demolition, a building's supports are knocked out and the building is broken up as it slams to the ground. In a conventional controlled-demolition, gravity is used to break up the building. Here, it seems that the only use of gravity was to get the dust out of the air.

Conclusion:

In conclusion, the explanations of the collapse that have been given by the 9/11 Commission Report and NIST are not physically possible. A new investigation is needed to determine the true cause of what happened to these buildings on September 11, 2001. The destruction of all seven WTC buildings and especially WTC1 and WTC2 may be considered the greatest engineering disaster in the history of the world and deserves a thorough investigation.

Top

Jesse Ventura on 9/11 with Alex Jones 2008
Part 1 of 3

Figure 9(b). Jesse Ventura has studied this page and knows these values. He also recognizes that the buildings were turned into powder.
(7:59) URL

(1:14 - 3:36) Jesse Ventura describes the various BBE cases. Impressive! Jessee Ventura nicely relayed the concepts presented above.
Note, the motion must restart after each floor because all kinetic energy has been consumed by failing the supports for each floor as well as pulverizing each floor. There wouldn't even be enough energy to do either, but assuming there had been enough energy, a time is calculated.

Appendix A: For those concerned about momentum.

Conservation of Momentum and Conservation of Energy

Conservation of Momentum:

The amount of momentum (p) that an object has depends on two physical quantities: the mass and the velocity of the moving object.

p = mv

where p is the momentum, m is the mass, and v the velocity.

If momentum is conserved it can be used to calculate unknown velocities following a collision.

(m_{1 *}v₁)_i + (m_{2 *}v₂)_i = (m_{1 *}v₁)_f + (m_{2 *}v₂)_f

where the subscript i signifies initial, before the collision, and f signifies final, after the collision.

If (m₁)_i = 0, and (v₂)_i = 0, then (v₂)_f must =0.
So, for conservation of momentum, there cannot be pulverization.

____________________________________

If we assume the second mass is initially at rest [(v₂)_i = 0], the equation reduces to

(m_{1 *}v₁)_i = (m_{1 *}v₁)_f + (m_{2 *}v₂)_f

As you can see, if mass m₁ = m₂ and they "stick" together after impact, the equation reduces to ,

(m_{1 *}v₁)_i = (2m_{1 *}v_new)_f

or v_new = (1/2) _*v₁

If two identical masses colliding and sticking together, they will travel at half the speed as the original single mass.

Conservation of Energy:

In elastic collisions, the sum of kinetic energy before a collision must equal the sum of kinetic energy after the collision. Conservation of kinetic energy is given by the following formula:

(1/2)(m_{1 *}v²₁)_i + (1/2)(m_{2 *}v²₂)_i = (1/2)(m_{1 *}v²₁)_f + (1/2)(m_{2 *}v²₂)_f + (Pulverize) + (Fail Floor Supports)

where (Pulverize) is the energy required to pulverize a floor and (Fail Floor Supports) is the energy required to fail the next floor.

If (1/2)(m_{1 *}v²₁)_i + (1/2)(m_{2 *}v²₂)_i = (Pulverize) + (Fail Floor Supports), there well be no momentum transfer.

In reality, (1/2)(m_{1 *}v²₁)_i + (1/2)(m_{2 *}v²₂)_i < (Pulverize) + (Fail Floor Supports),

So, for conservation of energy, we must assume there is some additional energy such that,

(1/2)(m_{1 *}v²₁)_i + (1/2)(m_{2 *}v²₂)_i + (Additional Energy) = (Pulverize) + (Fail Floor Supports),

where (Additional Energy) is the additional amount of energy needed to have the outcome we observed on 9/11/01.

Top

Appendix B: Assuming elastic collisions:

Assume that the top floor stays intact as a solid block weight, Block-A. Start the collapse timer when the 109th floor fails. At that instant, assume floor 108 miraculously turns to dust and disappears. So, Block-A can drop at free-fall speed until it reaches the 108th floor. After Block-A travels one floor, it now has momentum. If all of the momentum is transferred from Block-A to Block-B, the next floor, Block-A will stop moving momentarily, even if there is no resistance for the next block to start moving.

(m_{1 *}v₁)_i = (m_{2 *}v₂)_f

If Block-A stops moving, after triggering the next sequence, the mass of Block-A will not arrive in time to transfer momentum to the next "pancaking" between Block-B and Block-C. In other words, the momentum will not be increased as the "collapse" progresses.

However, as we can observe, the building disintegrated from the top down and there was no block of material.

Recall the physics demonstration shown below. (I believe everyone who has finished high school has seen one of these momentum demonstrations at some point in their life.)

(a) conservation of momentum if

no pulverization,
no structural resistance

(b) w/pulverization

there's no mass left to impact, so
there's no momentum to transfer


(c) Here, the building in each picture has lost more than half its height. But, where has this mass gone?


(d) disintegration of building during "collapse."


(e) The energy spent in the upward direction and the horizontal direction is no longer available to send material in the downward direction.

Figure 10. Images of (a)(b) Physics demonstration, (c)(d)(e) disintegration of building during "collapse."

So, if motion must be restarted at every floor, the total collapse time must be more than 10 seconds. Given that the building disintegrated from the top down, it is difficult to believe there could be much momentum to transfer, if any. Also, consider the energy required to pulverize the floor between each "pancake." After being pulverized, the surface-area/mass is greatly increased and the air resistance becomes significant. I don't believe this pulverized material can contribute any momentum as it "hangs" in the air and floats down at a much-much slower rate than the "collapsing" floors.

Consider reality:

Quiz #1:

QUESTIONS:
(1) How likely is it that all supporting structures on a given floor will fail at exactly the same time?
(2) If all supporting structures on a given floor did not fail at the same time, would that portion of the building tip over or fall straight down into its own footprint?
(3) What is the likelihood that supporting structures on every floor would fail at exactly the same time, and that these failures would progress through every floor with perfect symmetry and perfect timing?

Quiz #2:

Note, the alert observer will recognize that this analysis utilizes the conservation of momentum.
(For a review, see here and here and here and here and here.)

QUESTIONS -- for those who chose Model A instead of Model B:
(1) How did the volume of dust covering all of the streets in Manhattan (shown in Figure 12) contribute to a "collapse"?
(2) How did the volume of material shown squirting upward and outward (shown in Figure 13) contribute to a "collapse"?
(3) Where is the mass that you think pushed down on the building? Where are the "pancakes" in the photo shown in Figure 14 (which was taken around mid-day on 9/11/01)? Where are the "pancakes" in the photo shown in Figure 15?
(4) In Figure 15, the ambulance identifies ground level and was parked directly in front of WTC1. Where did the 110-story building go? Please identify where 110 stories worth of material is hiding in Figure 15.
(5) Figure 16 shows the two 110-story buildings before 9/11/01. Please explain where these 110-stories of material are in Figure 17.
(6) Figure 16 shows WTC6, WTC1, and WTC2 before 9/11/01. WTC1 (a 110-story building) is in the center of the photo and WTC6 (an 8-story building) is on the left, with yellow dimension lines showing its height. Please explain where the 110-stories of material from WTC1 are in Figure 18. Also, explain why there is a void in the middle of WTC6 in Figure 18. That is, if all of the material acted as a pile driver to push WTC1 down, why is the middle of WTC6 missing? And if part of WTC1 landed on WTC6 and made that hole, why is the hole empty?
(7) Figure 18 shows about eight stories of the north wall of WTC1 remaining. Where are the other 102 stories of outer wall?
(8) Figure 19 is a photo looking south, across the intersection of Vesey Street, and was taken shortly after WTC1 went away. WTC6 is on the left. The people in the photo have just emerged from their hiding places to find that WTC1 is no longer there. Please explain why there are no large solid sections of building visible in this photograph.


Model A		Model B
(a): The floors remain intact and pile up like a stack of pancakes, from the top down.		(b): The floors blow up like an erupting volcano from the top down
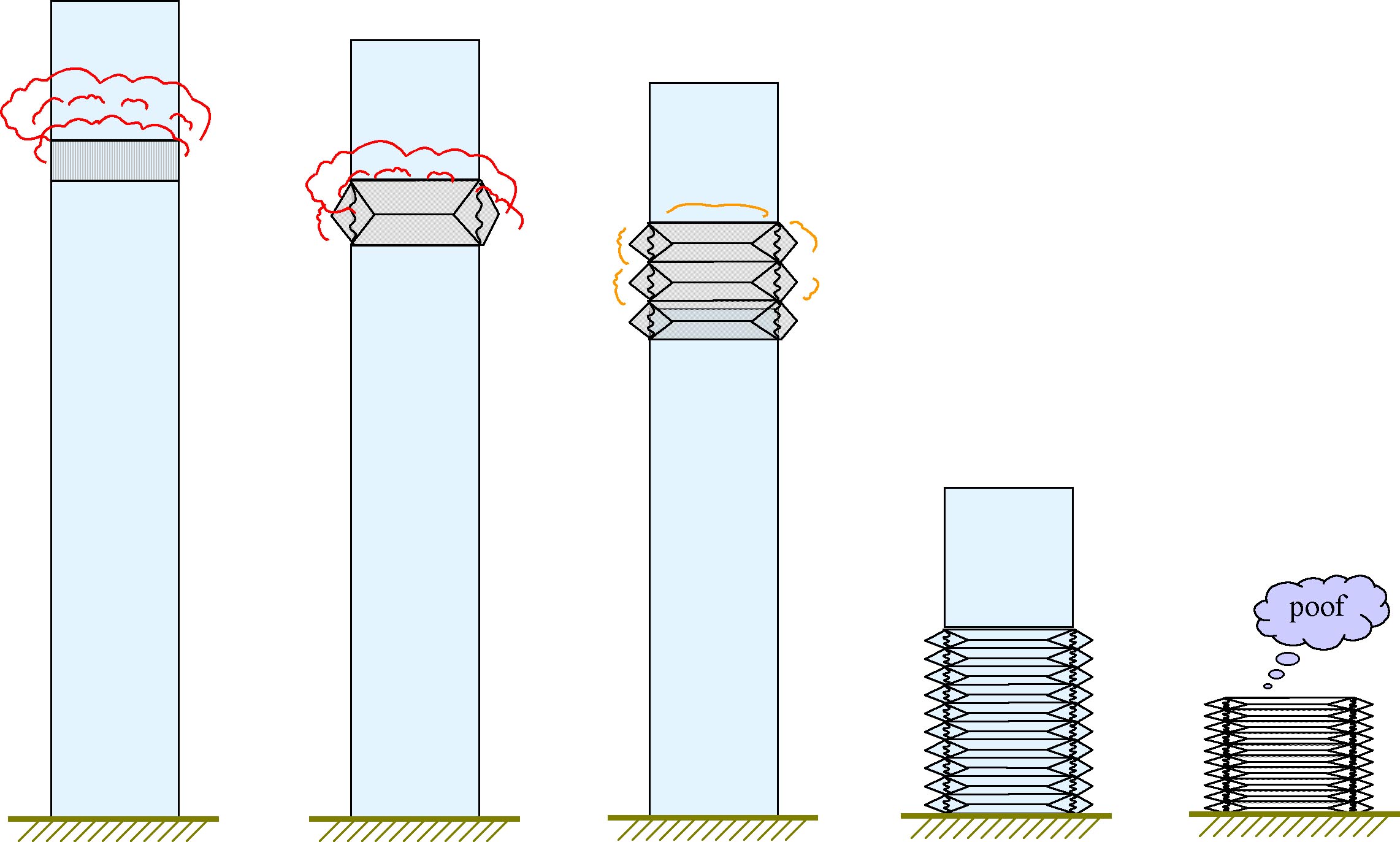
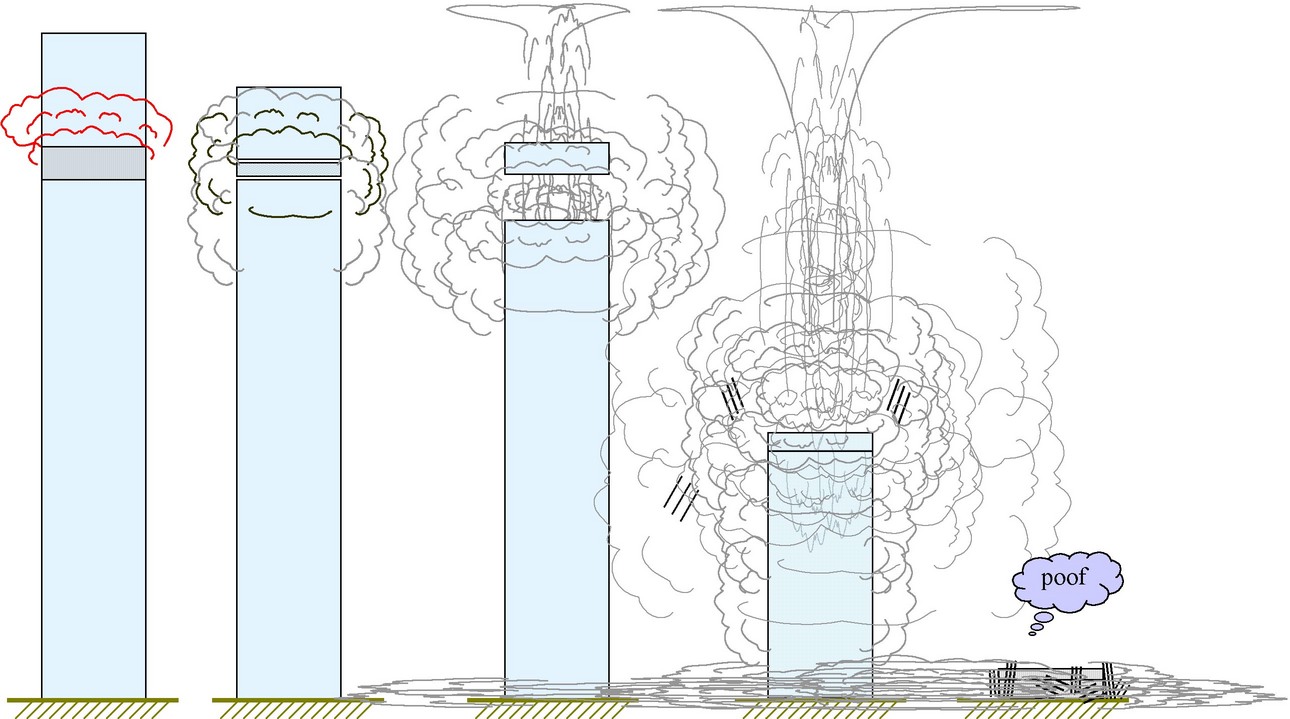
Figure 11: Choices to consider for modeling the "collapse." In order to choose Model A, all of the building material (which in this model is assumed to "push" the building down like a pile driver) should still be visible in the "footprint" of the building after the event. Now, let's look at the evidence, below.


Figure 12. A thick layer of uniform powder covered the streets of Manhattan after the destruction. This is next to the Fulton Street subway entrance, wich is several blocks east of the WTC. Church Street is in the distance.	Figure 13. The building turns to powder. Material is being launched upward and outward. The whitish material arcs over and begins to fall downward while the southern portion (away from the camera) appears to shoot straight up. (9/11/01) Cropped from Source

Figure 14. WTC6, an 8-story building, towers over the "rubble pile" remaining from WTC1 and 2. We know this photo was take around noon on 9/11/01. WTC7 can be seen in the distance, so we know what day this photo was taken. The Verizon Building is at a distance on the left. Where did the building go?

(9/11/01) Source and here

Figure 15. This is a view from West Street, looking east across the remains of WTC1. FEMA entered this photo on 9/13/01, which is the earliest date for any posts for the 9/11 event. Other photos they have for 9/13/01 show much more people and equipment present. So, it is believed that this photo was taken on 9/11/01, but entered into their files on 9/13. Where did the building go?
(9/13/01 entered, likely taken on 9/11/01) Source

Now you see it.	Now you don't.

Figure 16. The height of the towers is compared to the height of the 8-story WTC6 and the "rubble pile" shown in Figure 17. (1978) Source	Figure 17. This photo was taken was take around noon on 9/11/01, showing the height of WTC6 compared to the "rubble pile" of WTC1 which is appears in the foreground. Where did the building go? (9/11/01) Source and here	Figure 18. A view of the vacant lot where WTC1 stood just the day before. Where did the building go? (9/12/01)

Figure 19. Moments after WTC1 turns to powder, people emerge from their hiding places, looking amazed. From the postures, these folks look amazed. They are probably wondering if they are asleep and dreaming. After all, there should be a 110-story building directly in front of them. Where did it go? They are looking across the Vesey Street intersection at the remains of WTC6. In the distance, on the far side of the intersection, a fellow stands in amazement, with his hands on his hips. These people are approximatey 200 to 300 feet from where the 1,368-foot north wall of WTC1 stood. There appear to be no obvious indications that a 110-story building, over a quarter mile tall, had been there earlier in the day. Except for a few pieces of aluminum cladding strewn to the side, some paper and dust are all that remain. Where did the building go?
(9/11/01) Source

Quiz #3:

(1) What is the purpose of this webpage? (see here)
(2) Why are billiard balls used as timing devices? (see here)
(3) Don't you need to model what really happened in order to prove the official story false? (see here)
(4) If you wish to email me to insist this page is flawed, please include your answers to all of the above quiz questions so that I can better help you. Please include your answers to the three Quiz #1 questions, the eight Quiz #2 questions, and the three Quiz #3 questions above.

Top

The World Trade Center Towers as Bio-inspired Structures:
Characteristics of their Design and Demise

This work was presented at the 2006 Society for Experimental Mechanics Annual Conference
Adam’s Mark Hotel St. Louis, Missouri USA June 7, 2006
by Dr. Judy Wood, professor of Mechanical Engineering

The PowerPoint Presentation is provided here.

Shortcuts:
Jump to: *Case 1* Jump to: energy Jump to: remove Jump to: timing Jump to: *Case 2* Jump to: *Case 3* Jump to: *Case 4* Jump to: dust	Jump to: Jesse Ventura Video Jump to: Appendix A Jump to: Conservation of Momentum Jump to: Conservation of Energy Jump to: Appendix B Jump to: Quiz #1 Jump to: Quiz #2 Jump to: Quiz #3